Right Triangular Pyramid

 

surface area of a Right Triangular Pyramid formula
\( A_s \;= \;  \dfrac{1}{2} \cdot \left(h_b \cdot a\right) + \dfrac{3}{2} \cdot \left(a \cdot h_s\right)  \) 
Symbol English Metric
\( A_s \) = surface area \( in^2 \) \( mm^2 \)
\( a \) = edge \( in \) \( mm \)
\( h_b \) = height base \( in \) \( mm \)
\( h_s \) = height side \( in \) \( mm \)
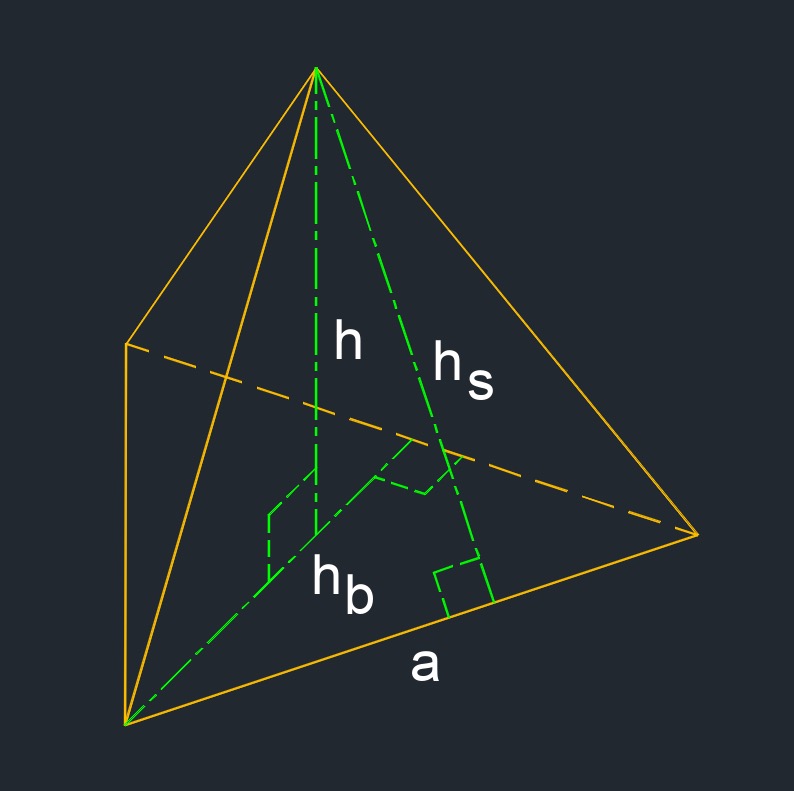  • right triangular pyramid 2Right triangular prism (a three-dimensional figure) has a triangle base and the apex alligned above the center of the base.
  • 1 base
  • 6 edges
  • 3 faces
  • 4 vertexs

Piping Designer Logo 1 

Volume of a Right Triangular Pyramid formulas

\( V \;=\;  \dfrac{1}{6} \cdot h_b \cdot a \cdot h \) 

\( V \;=\;  \dfrac{1}{3} \cdot A_b \cdot h \) 
Symbol English Metric
\( V \) = volume \( in^3 \) \( mm^3 \)
\( A_b \) = base area \( in^2 \) \( mm^2 \)
\( a \) = edge \( in \) \( mm \)
\( h \) = height \( in \) \( mm \)
\( h_b \) = height base \( in \) \( mm \)

right triangular pyramid 2