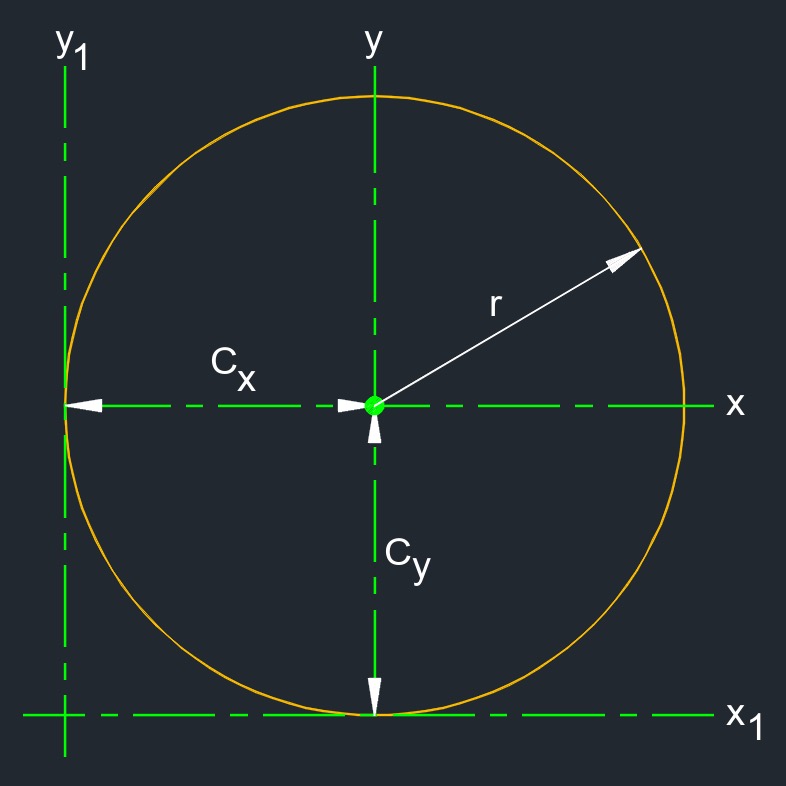
Radius of Gyration of a Circle formulas |
||
|
\( k_{x} \;=\; \dfrac{ r }{ 2 }\) \( k_{y} \;=\; \dfrac{ r }{ 2 }\) \( k_{z} \;=\; \dfrac{ \sqrt{2} }{ 2 } \cdot r \) \( k_{x1} \;=\; \dfrac{ \sqrt{5} }{ 2 } \cdot r \) \( k_{y1} \;=\; \dfrac{ \sqrt{5} }{ 2 } \cdot r \) \( k_{z1} \;=\; \dfrac{ \sqrt{10} }{ 2 } \cdot r \) |
||
| Symbol | English | Metric |
| \( k \) = radius of gyration | \( in \) | \( mm \) |
| \( r \) = radius | \( in \) | \( mm \) |
