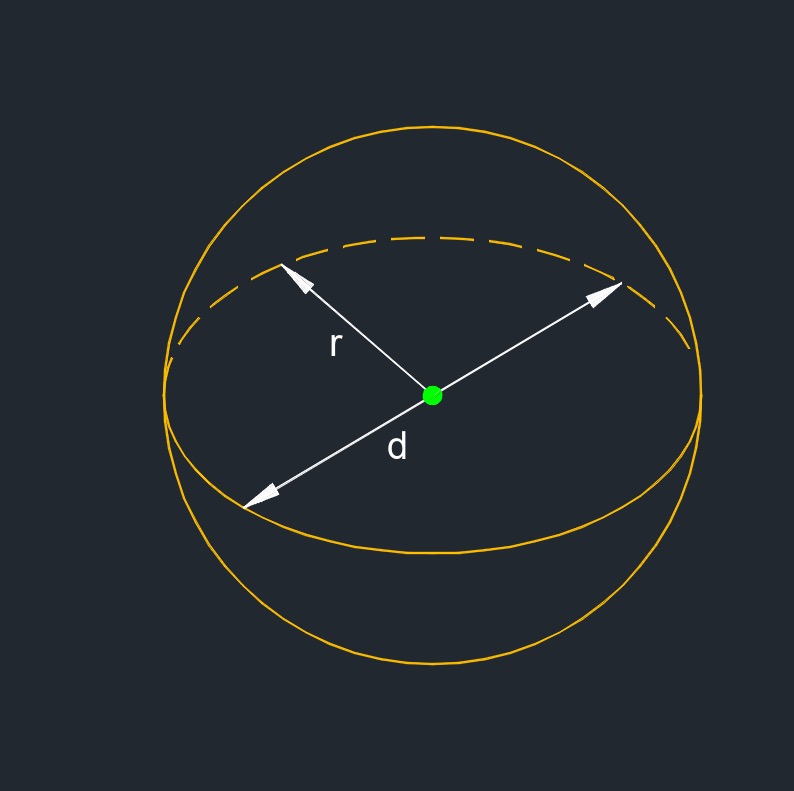
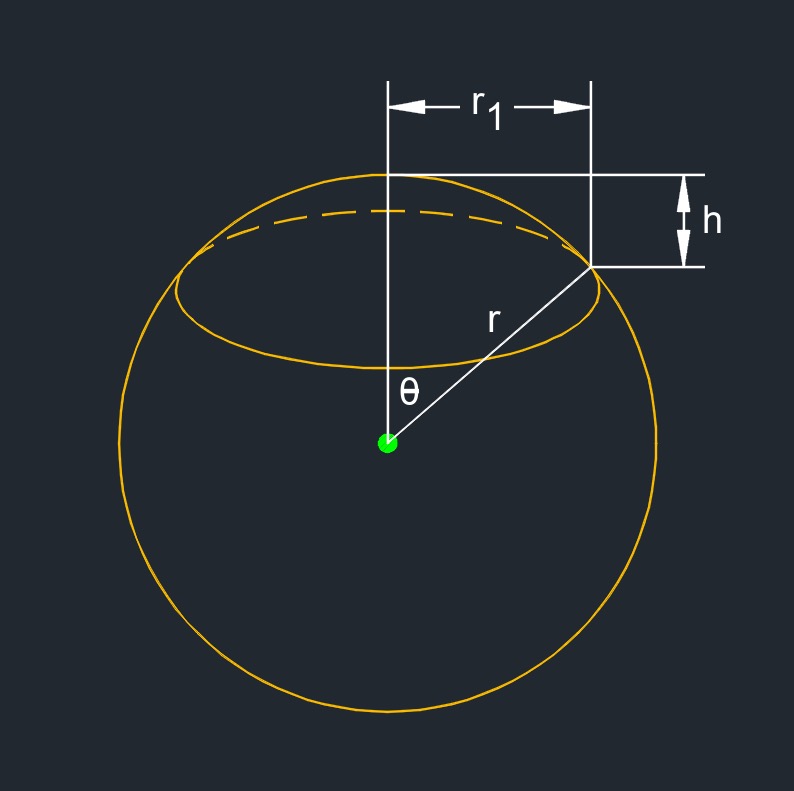
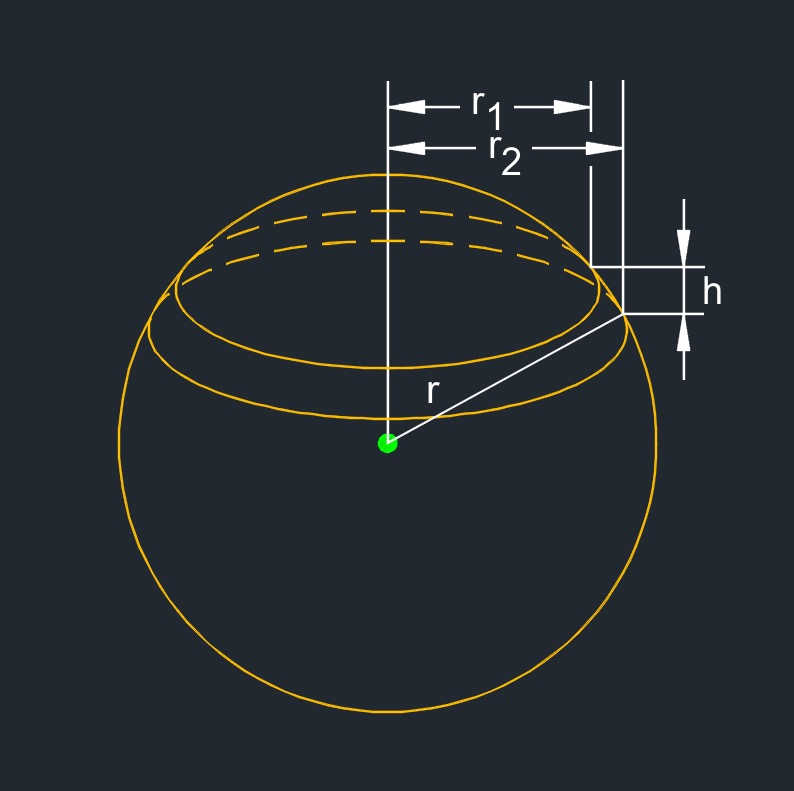
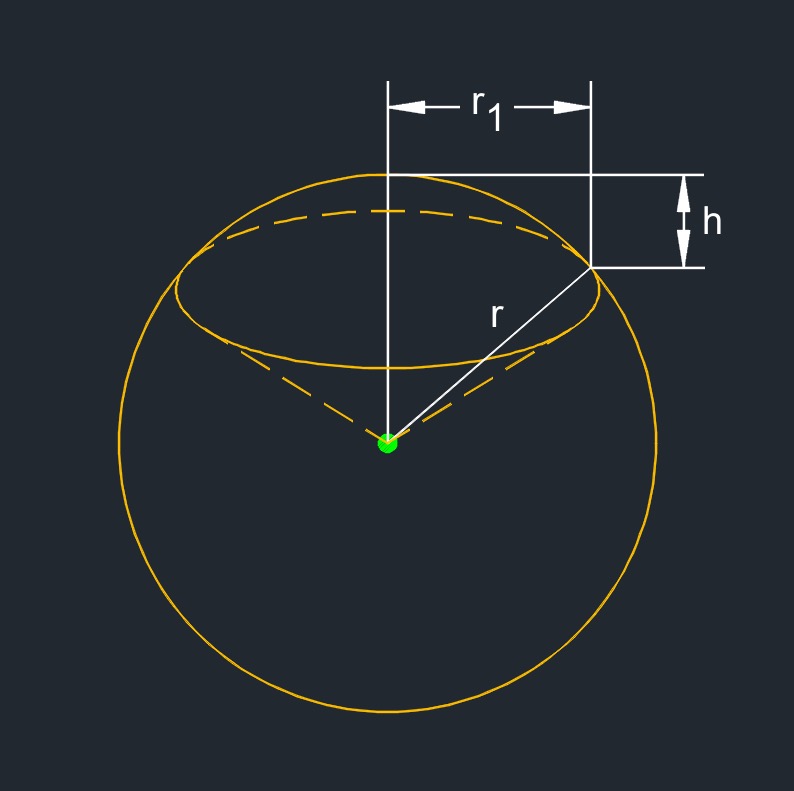
Sphere
- Sphere (a three-dimensional figure) has all points equally spaces from a given point of a three dimensional solid.
- Lune of a sphere is the space occupied by a wedge from the center of the sphere to the surface of the sphere.
- Sector of a sphere is the space occupied by a portion of the sphere with the vertex at the center and conical boundary.
- Segment and zone of a sphere is the space occupied by a portion of the sphere cut by two parallel planes.
- Sperical cap is the space occupied by a portion of the sphere cut by a plane.
Sphere Index
- Circumference of a Sphere
- Diameter of a Sphere
- Radius of a Sphere
- Surface Area of a Sphere
- Volume of a Sphere
- Surface Area of a Sphere Cap
- Volume of a Sphere Cap
- Surface Area of a Sphere Segment
- Volume of a Sphere Segment
- Surface Area of a Sphere Wedge
- Volume of a Sphere Wedge
- Volume of a Sphere Sector
Circumference of a Sphere formula |
||
| \( C = 2 \; \pi \; r \) | ||
| Symbol | English | Metric |
| \( C \) = circumference | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = radius | \( in \) | \( mm \) |
| \( d \) = diameter | \( in \) | \( mm \) |
Diameter of a Sphere formula |
||
| \( d = 2\;r \) | ||
| Symbol | English | Metric |
| \( d \) = diameter | \( in \) | \( mm \) |
| \( r \) = radius | \( in \) | \( mm \) |
RADIUS of a sphere formula |
||
|
\( r = \sqrt{ S \;/\; 4 \; \pi } \) \( r = \sqrt{ ( 3 \;/\; 4 ) \; ( V \;/\; \pi ) } \) |
||
| Symbol | English | Metric |
| \( r \) = radius | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( S \) = surface area | \( in^2 \) | \( mm^2 \) |
| \( V \) = volume | \( in^3 \) | \( mm^3 \) |
Surface Area of a sphere formula |
||
| \( S = 4\; \pi \;r^2 \) | ||
| Symbol | English | Metric |
| \( S \) = surface area | \( in^2 \) | \( mm^2 \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = radius | \( in \) | \( mm \) |
Volume of a sphere formula |
||
|
\( V = \frac{4}{3} \; \pi \;r^3 \) \( V = \pi \; d^3 \;/\; 6 \) |
||
| Symbol | English | Metric |
| \( V \) = volume | \( in^3 \) | \( mm^3 \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = radius | \( in \) | \( mm \) |
Surface Area of a sphere Cap formula |
||
| \( S = 2 \; \pi \; r \; h \) | ||
| Symbol | English | Metric |
| \( S \) = surface area | \( in^2 \) | \( mm^2 \) |
| \( h \) = height | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = radius | \( in \) | \( mm \) |
VOLUME of a sphere Cap formula |
||
|
\( V = \frac {1}{3} \; \pi\;h^2 \left( 3 r - h \right) \) \( V = \frac {1}{6} \; \pi\;h \left( 3 r_1{^2} + h^2 \right) \) |
||
| Symbol | English | Metric |
| \( V \) = volume | \( in^3 \) | \( mm^3 \) |
| \( h \) = height | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = radius | \( in \) | \( mm \) |
| \( r_1 \) = radius | \( in \) | \( mm \) |
Surface Area of a sphere Segment formula |
||
| \( S = 2 \; \pi \; r \; h \) | ||
| Symbol | English | Metric |
| \( S \) = surface area | \( in^2 \) | \( mm^2 \) |
| \( h \) = height | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = radius | \( in \) | \( mm \) |
VOLUME of a sphere Segment formula |
||
| \( V = \frac {1}{6} \; \pi\;h \left( 3 r_1{^2} + 3 r_2{^2} + h^2 \right) \) | ||
| Symbol | English | Metric |
| \( V \) = volume | \( in^3 \) | \( mm^3 \) |
| \( h \) = height | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = radius | \( in \) | \( mm \) |
| \( r_1 \) = radius | \( in \) | \( mm \) |
| \( r_2 \) = radius | \( in \) | \( mm \) |
Surface Area of a sphere WEDGE formula |
||
| \( S = \frac{ \theta }{ 360 } \; 4 \; \pi \; r^2 \) | ||
| Symbol | English | Metric |
| \( S \) = surface area | \( in^2 \) | \( mm^2 \) |
| \( \theta \) = angle | \( deg \) | \( rad \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = radius | \( in \) | \( mm \) |
Volume of a sphere WEDGE formula |
||
| \( V = \frac{ \theta }{ 2 \; \pi } \; \frac{ 4 }{ 3 } \; \pi \; r^2 \) | ||
| Symbol | English | Metric |
| \( V \) = volume | \( in^3 \) | \( mm^3 \) |
| \( \theta \) = angle | \( deg \) | \( rad \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = radius | \( in \) | \( mm \) |
VOLUME of a sphere SECTOR formula |
||
| \( V = \frac {2}{3}\; \pi \; r^2\;h \) | ||
| Symbol | English | Metric |
| \( V \) = volume | \( in^3 \) | \( mm^3 \) |
| \( h \) = height | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = radius | \( in \) | \( mm \) |
| \( r_1 \) = radius | \( in \) | \( mm \) |
