HomeMathematicsGeometrySolid GeometryCube
Print

Cube

on . Posted in Solid Geometry

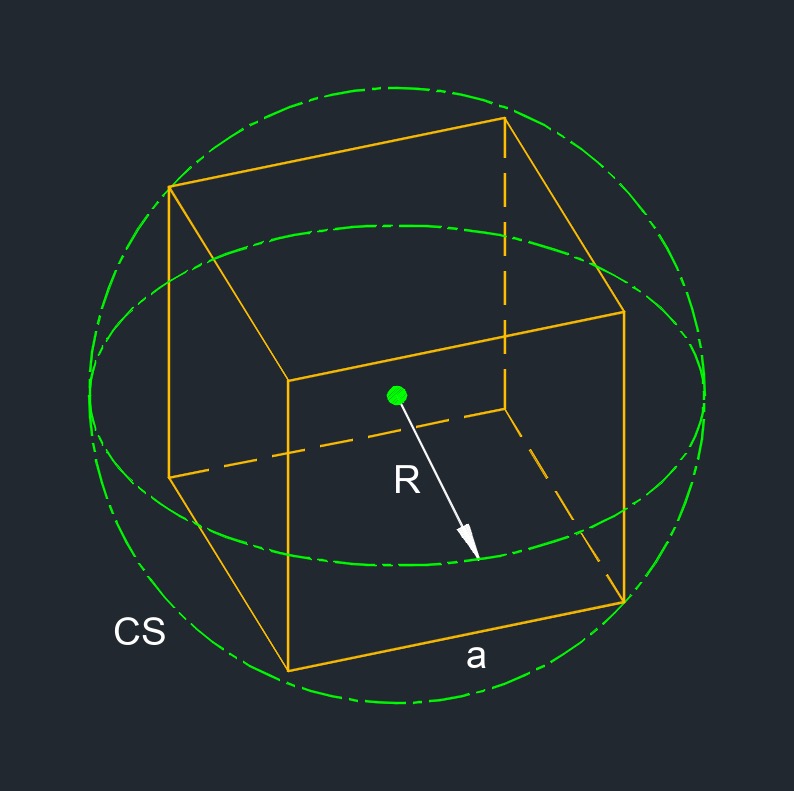
  • cube 10Cube (a three-dimensional figure) is a regular polyhedron with square faces.
  • All edges are the same length.
  • All faces are squares
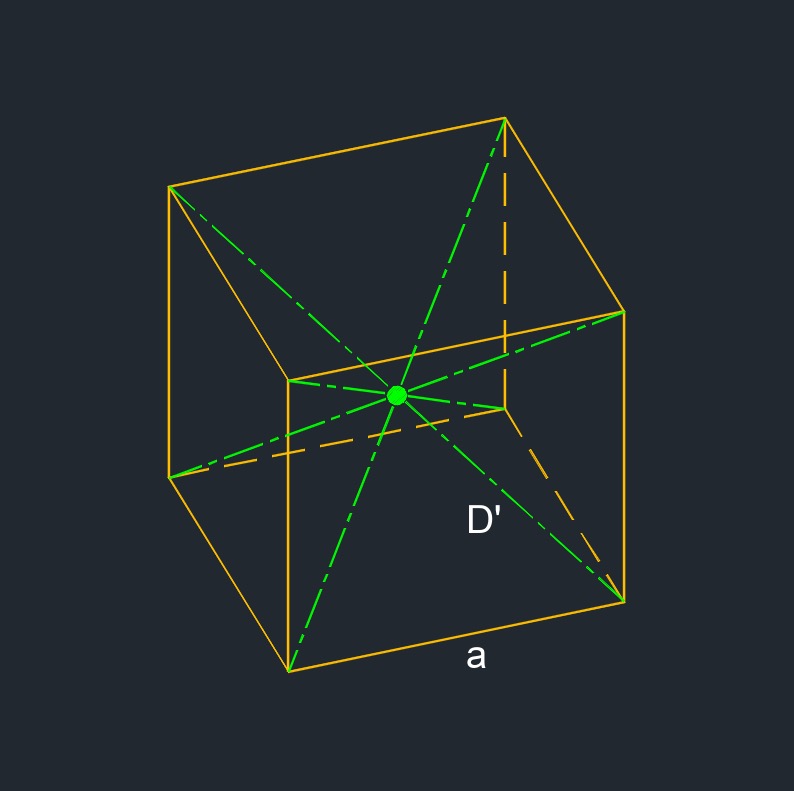
  • Diagonal is a line from one vertices to another that is non adjacent.
  • Circumscribed sphere is a polyhedron is a sphere that contains the polyhedron and touches each of the ployhedron's vertices.
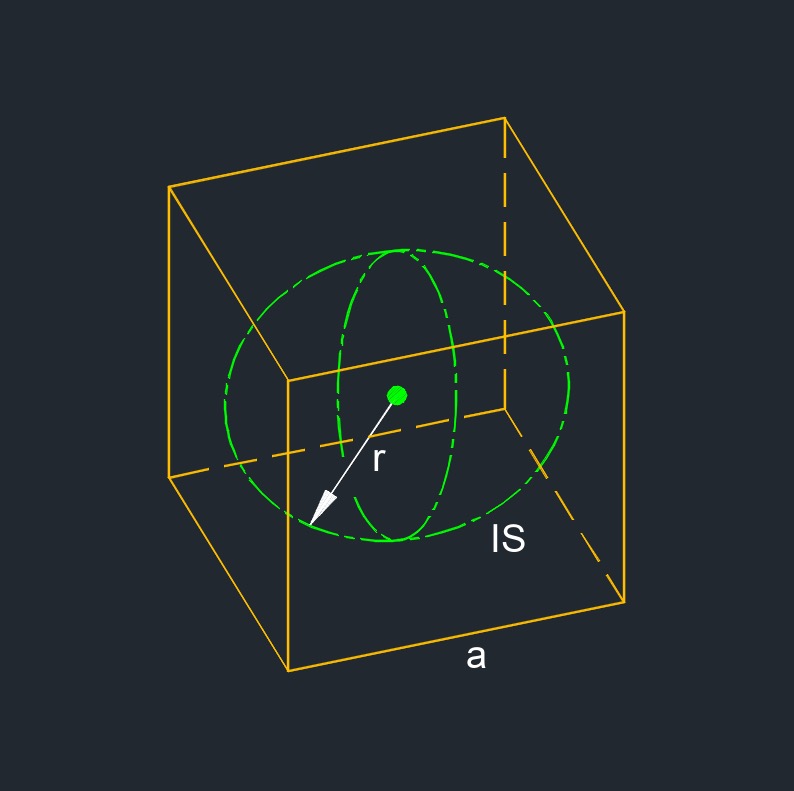
  • Inscribed sphere is a convex polyhedron is a sphere that is contained within the polyhedron and tangent to each of the polyhedron's faces.
  • Midsphere is a polyhedron is a sphere that is tangent to every edge of the polyhedron.
  • 4 base diagonals
  • 24 face diagonals
  • 4 space diagonals
  • 12 edges
  • 6 faces
  • 8 vertex

Cube Index

 

cube 7

Cube Circumscribed Sphere Radius formula

Circumscribed sphere is a polyhedron is a sphere that contains the polyhedron and touches each of the ployhedron's vertices.
\( R =  a \;  \sqrt {3} \;/\;2 \) 
Symbol English Metric
\( R \) = circumscribed sphere radius \( in \) \( mm \)
\( a \) = edge \( in \) \( mm \)

 

cube 7

Circumscribed Sphere Volume of a Cube formula

Circumscribed sphere is a polyhedron is a sphere that contains the polyhedron and touches each of the ployhedron's vertices.
\( C_v =  (3\;/\;4) \; \pi \; ( a\; \sqrt{3} \;/\;2 )^3  \) 
Symbol English Metric
\( C_v \) = circumscribed sphere volume \( in^3 \) \( mm^3 \)
\( a \) = edge \( in \) \( mm \)
\( \pi \) = Pi \(3.141 592 653 ...\)

 

cube 12

Edge of a Cube formulas

\( a =  \sqrt{ A_s \;/\; 6 } \) 

\( a = V^{1/3} \) 

\( a =   \sqrt{ 3 }  \;\; (D' \;/\; 3)  \) 
Symbol English Metric
\( a \) = edge \( in \) \( mm \)
\( D' \) = space diagonal \( in \) \( mm \)
\( A_s \) = surface face area \( in \) \( mm \)
\( V \) = volume \( in^3 \) \( mm^3 \)

 

cube 12

Face Area of a Cube formula
\( A_{area} = a^2 \) 
Symbol English Metric
\( A_{area} \) = face area \( in^2 \) \( mm^2 \)
\( a \) = edge \( in \) \( mm \)

 

 

 

cube 8

Inscribed Radius of a Cube formula

Inscribed sphere is a convex polyhedron is a sphere that is contained within the polyhedron and tangent to each of the polyhedron's faces.
\( r = a\;/\;2  \) 
Symbol English Metric
\( r \) = inside radius \( in \) \( mm \)
\( a \) = edge \( in \) \( mm \)

 

 

cube 8

Inscribed Sphere Volume of a Cube formula

Inscribed sphere is a convex polyhedron is a sphere that is contained within the polyhedron and tangent to each of the polyhedron's faces.
\( I_v =  (3\;/\;4) \; \pi \;  ( a \;/\;2 )^3  \) 
Symbol English Metric
\( I_v \) = inscribed sphere volume \( in^3 \) \( mm^3 \)
\( a \) = edge \( in \) \( mm \)
\( \pi \) = Pi \(3.141 592 653 ...\)

 

 

cube 9

Midsphere Radius of a Cube formula

Midsphere is a polyhedron is a sphere that is tangent to every edge of the polyhedron.
\( r_m =  (a\;/\;2)\; \sqrt {2}   \) 
Symbol English Metric
\( r_m \) = midsphere radius \( in \) \( mm \)
\( a \) = edge \( in \) \( mm \)


 

cube 6

Space Diagonal of a Cube formula
\( D' = \sqrt {3} \;a  \) 
Symbol English Metric
\( D' \) = space diagonal \( in \) \( mm \)
\( a \) = edge \( in \) \( mm \)

 

 

 

cube 12

Surface face Area of a Cube formula
\( A_s = 6\;a^2 \) 
Symbol English Metric
\( A_s \) = surface face area \( in^2 \) \( mm^2 \)
\( a \) = edge \( in \) \( mm \)

 

 

 

cube 12

Surface to volume ratio of a Cube formula
\( S_v = 6\;/\;a \) 
Symbol English Metric
\( S_v \) = surface to volume ratio \( in^3 \) \( mm^3 \)
\( a \) = edge \( in \) \( mm \)

 

 

 

cube 12

Volume of a Cube formula
\( V =   a^3 \) 
Symbol English Metric
\( V \) = volume \( in^3 \) \( mm^3 \)
\( a \) = edge \( in \) \( mm \)

 

 

 

 

cube 12

Weight of a Cube formula
\( m =   a^3 \; \rho \) 
Symbol English Metric
\( m \) = mass \( lbm \)  \( kg \) 
\( \rho \)   (Greek symbol rho) = density \(lbm\;/\;ft^3\) \(kg\;/\;m^3\)
\( a \) = edge \( in \) \( mm \)

 

Piping Designer Logo 1

 

 

 

Tags: Volume Solid Prism

Latest Articles