Elastic Modulus of Concrete Formula |
||
|
\( E_c \;=\; n \cdot \sqrt{ f_{c}{'} } \) (Elastic Modulus of Concrete) \( n \;=\; \sqrt{ \dfrac{ E_c^2 }{ f_{c}{'} } } \) \( f_{c}{'} \;=\; \dfrac{ E_c^2 }{ n^2 }\) |
||
| Symbol | English | Metric |
| \( E_c \) = Elastic Modulus of Concrete | \(lbf \;/\; in^2\) | \(MPa\) |
| \( n \) = Number (English units (57,000), Metric units (4700) | \(dimensionless\) | \( dimensionless \) |
| \( f_{c}{'} \) = Concrete Specified Cylinder 28-day Compression Strength | \(lbf \;/\; in^2\) | \(MPa\) |
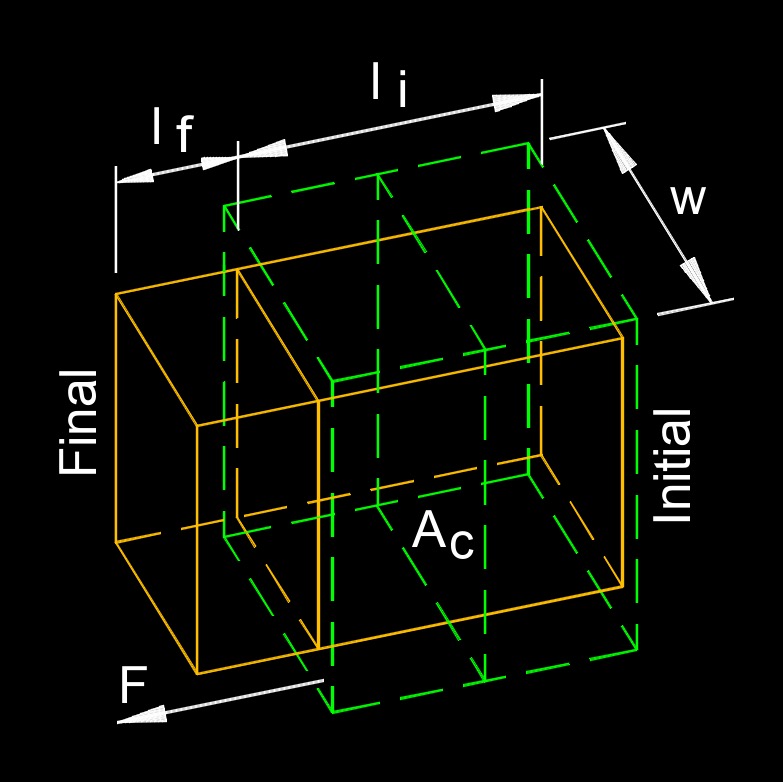Elastic modulus of concrete, abbreviated as \(E_c\), is valid for normal weight, normal density concrete. Concrete is not an elastic material, it will not recover its original shape on unloading. The modulus of elasticity is dependent on the compressive strength of concrete, properties of the coarse aggregates, the proportion of the aggregates in the concrete, quality of cement paste and addition of mineral admixtures.
Young's modulus of elasticity measures the stiffness of an elastic material. The ratio of the longitudinal stress applied to a body or substance to the resulting longitudinal strain within the elastic limits. The elastic modulus of concrete is a very important mechanical parameter reflecting the ability of the concrete to deform elastically. In order to make full use of the compressive strength potential, the structures using high-strength concrete tend to be slimmer and require a higher elastic modulus so as to maintain its stiffness.
